Natural Resource BiometricsStratified SamplingStratification for sampled areas is one of the most useful techniques in resource sampling. Stratification should be applied when a difference in the statistic of interest is expected to vary by the sampled strata. If this is true stratification can:
For example consider the following area in Figure 1: 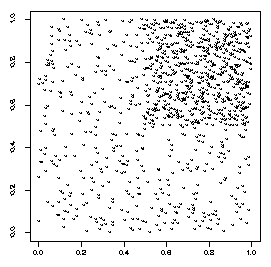 Figure 1. Population with potential strata If this population were sampled for density, without considering the differences in the regions of the population the estimate of variance will be higher. If this area were stratified, then sampled by strata, the overall estimate will have lower variance or few samples may be required. Consider the following histogram of a population that was generated from two normal distributions (Sample 1 mu=4, sigma=2; and Sample 2 mu=10, sigma=1) (see figure 2). The sample means are plotted as solid lines. The overall means are thicker lines than the two strata mean lines. The sample standard deviations (s) are plotted as dashed line is with the overall line thicker that the strata lines. This graph illustrates the increase in sample standard deviation because of pooling the two strata versus calculating the strata individually. 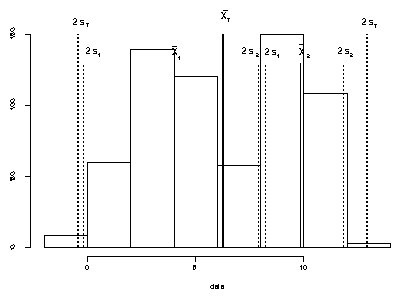 Figure 2. Example of pooling two strata Table 1. Simple Statistics for the two populations.
Estimate of the mean per stratum: 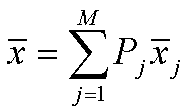
Estimate of the mean for the population: 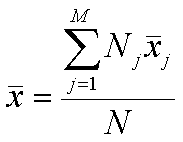
Estimate of the total for X for the entire population: 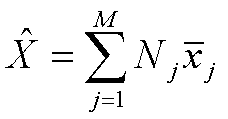
Variance of the mean for the population: 
If the strata are sufficiently large you can use this form to estimate variance: 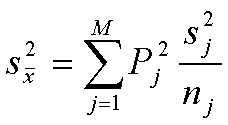
The standard error of the total estimate of x: 
Given these definitions: M = number of strata in the population n = total number of sampling units measured for all strata nj = total number of sampling units measured in the jth stratum N = total number of sampling units in the population Nj = total number of sampling units in the jth stratum Xij = quantity X measured on the ith sampling unit of the jth stratum
Pj = proportion of the total area in the jth stratum
Example SpreadsheetWeighted mean example spreadsheet
Also See: Chapter 12 - Sampling in Forest Inventory pages 156-192, in: Husch, B., T. W. Beers and J. A. Kershaw, Jr.. 2003. Forest Mensuration. Fourth Edition. John Wiley and Sons, Hoboken, New Jersey 443 p. Chapter 6 - Sample Designs - Random Sampling pages 200-236, in: Krebs, C. J. 1998. Ecological Methodology. Harper and Row, Publishers. New York. 620 pp. |

Natural Resources Biometrics by David R. Larsen is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License . Author: Dr. David R. Larsen Created: October 15, 2000 Last Updated: July 23, 2018 |