Line Transect

Hayne's Estimator

The Hayne estimator was develop to estimate density of birds that flush as an observer comes within a certain radius.  This method assumes that there is a fixed flushing distance r  and all animals within r distance of the observer will flush.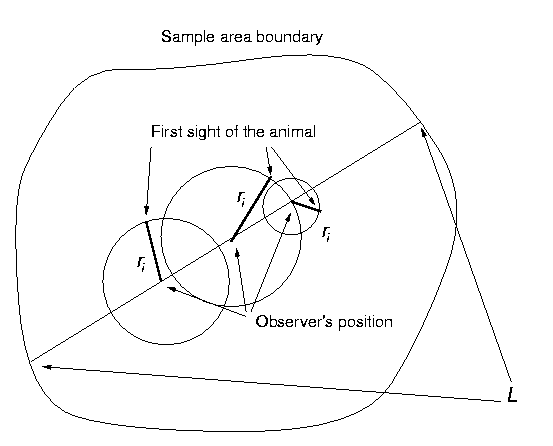

Figure 1: Illustrating the layout and important measures for the Hayne line transect estimator

The density is estimated by :

$$ \hat{D}_H = \frac{n}{2L} \left( \frac{1}{n} \sum_{i=1}^n \frac{1}{r_i} \right) $$

where:

    DH is the Hayne density estimate
    n is the number of animals
    L is the length of the transect
    ri is the sighting distance to the ith animal.
     

R is the mean of the reciprocal of the sighting distances and is calculated as:

$$ R = \frac{1}{n} \sum_{i=1}^n \frac{1}{r_i} $$

The variance of the density estimate is calculated as :

$$ Variance(\hat{D}_H = D_H^2 \left[ \frac{var(n)}{n^2} + \frac{\sum_{i=1}^n \left( \frac{1}{r_i} - R \right)^2}{R^2n(n-1)} \right] $$

where:

    DH is the Hayne density estimate

    n is the number of animals

    var(n) is the variance of n approximately equal to n

    ri is the sighting distance to the ith animal.

     

The standard error of the mean density is estimated by the square root of the variance.

Hayne method assumed that the mean sighing angle is 32.7o.  This can be tested by:

$$ Z = \frac{\sqrt{n} (\bar{\theta} - 32.7)}{21.56} $$
where:
    Z is the standard normal deviate test value
    n is the number of animals sighted
    Theta is the mean observed sighting angle (Figure 1).
     

The test would be if the Z value is greater than 1.96 or less than -1.96 the sighting angle is statistically different than 32.7o at the alpha = 0.05 level.


Also See:

Chapter 2 - Estimating Abundance: Line Transects pages 115-121 in:

Krebs, C. J. 1989. Ecological Methodology. Harper and Row, Publishers. New York. 654 pp.

Creative Commons License
Natural Resources Biometrics by David R. Larsen is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License .

Author: Dr. David R. Larsen
Created: November 6, 1998
Last Updated: December 14, 2019